How to Draw Number Lines for Fractions

Learning Outcomes

• Locate and label improper and proper fractions on a number line
• Order fractions and mixed numbers on a number line
• Use inequality symbols to compare fractions and mixed numbers

Now we are ready to plot fractions on a number line. This will help us visualize fractions and understand their values.

Doing the Manipulative Mathematics activity "Number Line Part [latex]3[/latex] " will help you develop a better understanding of the location of fractions on the number line.
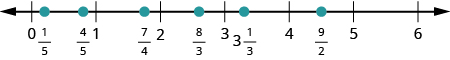
Let us locate [latex]\frac{1}{5},\frac{4}{5},3,3\frac{1}{3},\frac{7}{4},\frac{9}{2},5[/latex], and [latex]\frac{8}{3}[/latex] on the number line.
We will start with the whole numbers [latex]3[/latex] and [latex]5[/latex] because they are the easiest to plot.

The proper fractions listed are [latex]\frac{1}{5}[/latex] and [latex]\frac{4}{5}[/latex]. We know proper fractions have values less than one, so [latex]\frac{1}{5}[/latex] and [latex]\frac{4}{5}[/latex] are located between the whole numbers [latex]0[/latex] and [latex]1[/latex]. The denominators are both [latex]5[/latex], so we need to divide the segment of the number line between [latex]0[/latex] and [latex]1[/latex] into five equal parts. We can do this by drawing four equally spaced marks on the number line, which we can then label as [latex]\frac{1}{5},\frac{2}{5},\frac{3}{5}[/latex], and [latex]\frac{4}{5}[/latex].
Now plot points at [latex]\frac{1}{5}[/latex] and [latex]\frac{4}{5}[/latex].

The only mixed number to plot is [latex]3\frac{1}{3}[/latex]. Between what two whole numbers is [latex]3\frac{1}{3}?[/latex] Remember that a mixed number is a whole number plus a proper fraction, so [latex]3\frac{1}{3}>3[/latex]. Since it is greater than [latex]3[/latex], but not a whole unit greater, [latex]3\frac{1}{3}[/latex] is between [latex]3[/latex] and [latex]4[/latex]. We need to divide the portion of the number line between [latex]3[/latex] and [latex]4[/latex] into three equal pieces (thirds) and plot [latex]3\frac{1}{3}[/latex] at the first mark.

Finally, look at the improper fractions [latex]\frac{7}{4},\frac{9}{2}[/latex], and [latex]\frac{8}{3}[/latex]. Locating these points will be easier if you change each of them to a mixed number.

[latex]\frac{7}{4}=1\frac{3}{4},\frac{9}{2}=4\frac{1}{2},\frac{8}{3}=2\frac{2}{3}[/latex]
Here is the number line with all the points plotted.

Example

Locate and label the following on a number line: [latex]\frac{3}{4},\frac{4}{3},\frac{5}{3},4\frac{1}{5}[/latex], and [latex]\frac{7}{2}[/latex].

Solution:
Start by locating the proper fraction [latex]\frac{3}{4}[/latex]. It is between [latex]0[/latex] and [latex]1[/latex]. To do this, divide the distance between [latex]0[/latex] and [latex]1[/latex] into four equal parts. Then plot [latex]\frac{3}{4}[/latex].

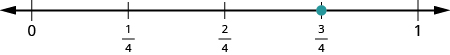
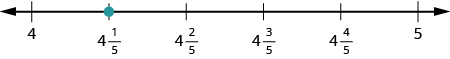
Next, locate the mixed number [latex]4\frac{1}{5}[/latex]. It is between [latex]4[/latex] and [latex]5[/latex] on the number line. Divide the number line between [latex]4[/latex] and [latex]5[/latex] into five equal parts, and then plot [latex]4\frac{1}{5}[/latex] one-fifth of the way between [latex]4[/latex] and [latex]5[/latex] .

Now locate the improper fractions [latex]\frac{4}{3}[/latex] and [latex]\frac{5}{3}[/latex] .
It is easier to plot them if we convert them to mixed numbers first.

[latex]\frac{4}{3}=1\frac{1}{3},\frac{5}{3}=1\frac{2}{3}[/latex]
Divide the distance between [latex]1[/latex] and [latex]2[/latex] into thirds.

Next let us plot [latex]\frac{7}{2}[/latex]. We write it as a mixed number, [latex]\frac{7}{2}=3\frac{1}{2}[/latex] . Plot it between [latex]3[/latex] and [latex]4[/latex].

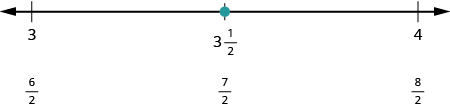
The number line shows all the numbers located on the number line.

Watch the following video to see more examples of how to locate fractions on a number line.

In Introduction to Integers, we defined the opposite of a number. It is the number that is the same distance from zero on the number line but on the opposite side of zero. We saw, for example, that the opposite of [latex]7[/latex] is [latex]-7[/latex] and the opposite of [latex]-7[/latex] is [latex]7[/latex].

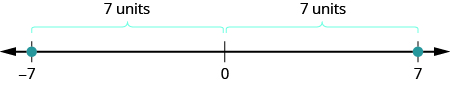
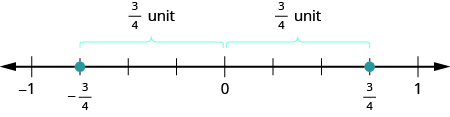
Fractions have opposites, too. The opposite of [latex]\frac{3}{4}[/latex] is [latex]-\frac{3}{4}[/latex]. It is the same distance from [latex]0[/latex] on the number line, but on the opposite side of [latex]0[/latex].

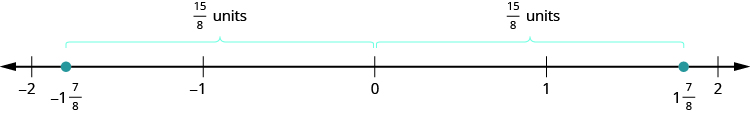
Thinking of negative fractions as the opposite of positive fractions will help us locate them on the number line. To locate [latex]-\frac{15}{8}[/latex] on the number line, first think of where [latex]\frac{15}{8}[/latex] is located. It is an improper fraction, so we first convert it to the mixed number [latex]1\frac{7}{8}[/latex] and see that it will be between [latex]1[/latex] and [latex]2[/latex] on the number line. So its opposite, [latex]-\frac{15}{8}[/latex], will be between [latex]-1[/latex] and [latex]-2[/latex] on the number line.

Example

Locate and label the following on the number line: [latex]\frac{1}{4},-\frac{1}{4},1\frac{1}{3},-1\frac{1}{3},\frac{5}{2}[/latex], and [latex]-\frac{5}{2}[/latex].

In teh next video we give more examples of how to locate negative and positive fractions on a number line.

Order Fractions and Mixed Numbers

We can use the inequality symbols to order fractions. Remember that [latex]a>b[/latex] means that [latex]a[/latex] is to the right of [latex]b[/latex] on the number line. As we move from left to right on a number line, the values increase.

Example

Order each of the following pairs of numbers, using [latex]<[/latex]; or [latex]>:[/latex]

1. [latex]-\frac{2}{3}[/latex] __ [latex]- 1[/latex]
2. [latex]-3\frac{1}{2}[/latex] __ [latex]- 3[/latex]
3. [latex]-\frac{3}{7}-[/latex] __ [latex]\frac{3}{8}[/latex]
4. [latex]-2[/latex] __ [latex]\frac{-16}{9}[/latex]

In the following video we show another example of how to order integers, fractions and mixed numbers using inequality symbols.

How to Draw Number Lines for Fractions

Source: https://courses.lumenlearning.com/prealgebra/chapter/locating-and-ordering-fractions-and-mixed-numbers-on-the-number-line/

Share this post